1 Answer

Vatavale · Answer 1 · 2023-04-21T13:50:36+0000

We have to find the value of z.

We know that the surface area is 378 ft².

We can then write the expression for the surface area. It will be the sum of the areas of each face.

There are 6 faces, that can be grouped in 3 pairs of faces. Each pair has an area that is the product of the length and width of each face.

Then, we can write the surface area as:

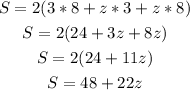
$\begin{gathered} S=2(3*8+z*3+z*8) \\ S=2(24+3z+8z) \\ S=2(24+11z) \\ S=48+22z \end{gathered}$

As S = 378, we can find z as:

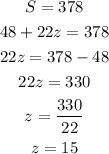
$\begin{gathered} S=378 \\ 48+22z=378 \\ 22z=378-48 \\ 22z=330 \\ z=(330)/(22) \\ z=15 \end{gathered}$

Answer: z = 15

Please log in or register to add a comment.