Question

A right triangular pyramid has a 12-meter height and a base with legs that are 3meters and 4 meters long. Find the volume of the triangular pyramid.O 24 m3O 144 m3O 72 m30 48 m3

Answer 1

`A)24m^3`

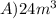
Step-by-step explanation

We want to find the volume of the right triangular pyramid given.

The volume of a pyramid is given as:

`V=(1)/(3)\cdot B\cdot h`

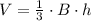
where B = base area; h = height

We have to first find the area of the base, B.

The base is a right-angle triangle with legs 3 meters and 4 meters. Let us make a quick sketch of the triangle:

Therefore, we can find the area of this triangle using:

`B=(1)/(2)\cdot b\cdot h`

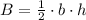
where b = base length = 3 m

h = height = 4 m

Therefore, we have that:

`\begin{gathered} B=(1)/(2)\cdot4\cdot3 \\ B=6m^2 \end{gathered}`

Hence, the volume of the right triangular pyramid is:

`\begin{gathered} V=(1)/(3)\cdot12\cdot6 \\ V=24m^3 \end{gathered}`

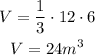
The answer is Option A.