Question

I am having difficulty solving derivatives. I was wondering if you could explain the product and quotient rule with examples? Thank you in advance!

Answer 1

Given:

Product and quotient rule.

Required:

Explain the product and quotient rule with examples.

Step-by-step explanation:

Product rule:

If two functions are in the multiple forms then the derivative of two functions is given by the formula:

`(d)/(dx)(u.v)=v(d)/(dx)u+u(d)/(dx)v`

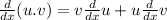
where u = first function and v = second function.

Example

`\begin{gathered} (d)/(dx)(x.sinx)=sinx.(d)/(dx)(x)+x(d)/(dx)(sinx) \\ (d)/(dx)(x.sinx)=sinx.(1)+x.(cosx) \\ (d)/(dx)(x.sinx)=sinx+xcosx \end{gathered}`

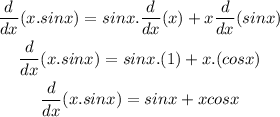
Quotient Rule:

If two functions are given in the quotient form or division form then the derivative of these functions using the quotient rule is given as:

`(d)/(dx)((u)/(v))=(v(d)/(dx)u-u(d)/(dx)v)/(v^2)`

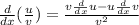
Example:

`\begin{gathered} (d)/(dx)((sinx)/(x))=(x(d)/(dx)sinx-sinx(d)/(dx)x)/(x^2) \\ (d)/(dx)((sinx)/(x))=(x(cosx)-sinx(1))/(x^2) \\ (d)/(dx)((sinx)/(x))=(xcosx-sinx)/(x^2) \end{gathered}`

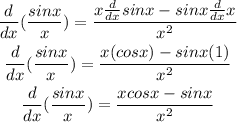
Final Answer:

As explained in the explanation part.