Step-by-step explanation
Step 1
find the vertex,
when you have a equation in the standard form

the vertex is given by

hence,
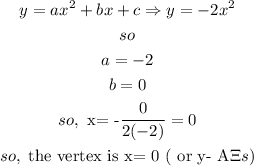
evaluate when x= 0 to find the coordinate of the vertex
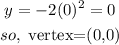
P1(0,0)
Step 2
now, 2 poins to the left
a)
for x=-1
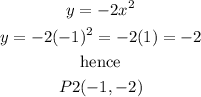
P2(-1,-2)
b)when x=-2

Step 3
2 points to the rigth
a)
for x=1
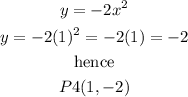
P4(1,-2)
b)when x=2
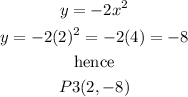
Step 4
I hope this helps you