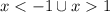Question

Solve the inequality x > 2 - |2x-1|​

Answer 1

`x <-1 \cup x>1`

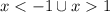
Explanation:

It can be solved analytically or graphically. Graphically is the fastest way if you know how to handle the two lines. Let's rearrange terms in either case:

`x-2>-|2x-1| \rightarrow |2x-1| >2-x`

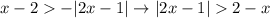
Graphic: Red line is
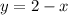`y=2-x`, solid blue line is
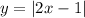`y=|2x-1|`, the thin extension is the parent line without the absolute value.

Solving the equation is equivalent to answer "when is the blue line higher than the red one?" Which obviously happens outside the two intercept points. Let's find the first coordinate of both - we don't really care what's the y coordinate of either point.

For the left point, the x coordinate is given by
`1-2x = 2-x \rightarrow 2x-1=x-2 \rightarrow x=-1`

For the right point, we have

`2x-1=2-x \rightarrow 3x=3 \rightarrow x=1`

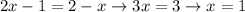
Analytical:

We split the problem in two parts: while the content absolute value is non negative, we have

`2x-1 \geq 0\\2x-1 >2-x\\\\x\geq \frac12\\3x > 3 \rightarrow x>1`

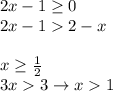
Which admits as solutions all valeus of x greater than 1 (since both have to be satisfied at once, and values between 1/2 and 1 will not work for the second.

OR, with a negative quantity inside the absolute value, we get

`2x-1 <0\\1-2x > 2-x\\\\x<\frac12\\x<-1`

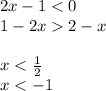
which again is satisfied by all values of x less than -1.

Thus the solution is given by
`x <-1 \cup x>1`