Question

You measure 26 dogs' weights, and find they have a mean weight of 59 ounces. Assume the population standard deviation is 14 ounces. Based on this, construct a 99% confidence interval for the true population mean dog weight.Give your answers as decimals, to two places < μ < ounces

Answer 1

Given that the mean weights of 26 dogs is 59 ounces, and the standard deviation is 14 ounces, this implies that

`\begin{gathered} sample\text{ mean = }\mu=59 \\ standard\text{ deviation = }\sigma=14 \\ sample\text{ size = }n=26 \end{gathered}`

If the confidence level is 99%, this implies that the critical value is evaluated as

`z_=2.567`

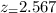
The margin of error is expressed as

`E=z*(\sigma)/(√(n))`

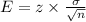
thus, the margin of error is evaluated to be

`\begin{gathered} E=2.567*(14)/(√(26)) \\ \Rightarrow E=7.048021665 \end{gathered}`

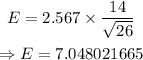
Hence, the limits of confidence interval are given by:

H