Substitute the coordinates of each point into the expression on the left hand side of the equation to find which of them make the expression to be equal to 4.

A (3,8)
Substitute x=3 and y=8:
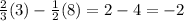
B (0,8)
Substitute x=0 and y=8:
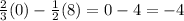
C (2,5)
Substitute x=2 and y=5:
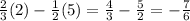
D (6,0)
Substitute x=6 and y=0:

Therefore, the point (6,0) is a solution to the given equation (option D).