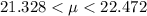Question

Assume that a sample is used to estimate a population mean u. Find the 80% confidence interval for a sampleof size 541 with a mean of 21.9 and a standard deviation of 10.4. Enter your answer as a tri-linear inequalityaccurate to 3 decimal places.< I

Answer 1

Given that the sample has the following information;

We can use the formula below to find the confidence interval;

`\mu\pm z(\frac{\sigma}{\sqrt[]{n}})`

The 80% confidence interval for a sample is usually given to be 1.28

We can then substitute all the values into the formula above.

`\begin{gathered} 21.9\pm1.28(\frac{10.4}{\sqrt[]{541}}) \\ 21.9\pm0.572 \end{gathered}`

We would then have the answer finalized as

Answer:

`21.328<\mu<22.472`