1 Answer

Iesus · Answer 1 · 2023-04-05T12:01:49+0000

Answer:

nπ /11

Step-by-step explanation:

To find the discontinuities of the function, we have to find the points where it is undefined.

Now

$8\cdot\csc 11x=8\cdot(1)/(\sin11x)$

therefore, the function is undefined where

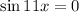
$\sin 11x=0$

because then

$8\cdot(1)/(\sin11x)=8\cdot(1)/(0)=\text{undefined}$

The sine function is zero wherever the angle is a multiple of pi:

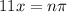
$11x=n\pi$

solving for x gives

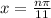
$x=(n\pi)/(11)$

which is our answer!

Please log in or register to add a comment.