Question

Find the area of the trapezoid plotted below.Round your answer to the whole number.

Answer 1

The area of the trapezoid is 10 squared units

Here, we want to find the area of the trapezoid

Mathematically, we have this as;

`A\text{ = }(1)/(2)(a+b)h`

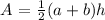
a and b represents the parallel bases, h represents the height

LH is a; the distance here is between (-6,-4) and (-4,-5)

WO is the distance b and the distance is between (-7,-1) and ( -1,-4)

h is the distance SH and it is between (-5,-2) and (-6,-4)

To find the distance between each of the points, we use the distance formula;

We have this as;

`\begin{gathered} D\text{ = }\sqrt[]{(x_2-x_1)^2+(y_2-y_1)^2} \\ \text{for LH;} \\ D\text{ = }\sqrt[]{(2)^2+(-1)^2}\text{ = }\sqrt[]{5} \\ \text{for WO;} \\ D\text{ = }\sqrt[]{6^2+3^2}\text{ =3 }\sqrt[]{5} \\ \text{for SH;} \\ D\text{ = }\sqrt[]{1^2+2^2}\text{ = }\sqrt[]{5} \end{gathered}`

Thus, we substitute these values and get;

`\begin{gathered} A\text{ = }(1)/(2)(\sqrt[]{5\text{ }}+3\sqrt[]{5})\sqrt[]{5} \\ \\ A\text{ = }\frac{4\sqrt[]{5}}{2}*\sqrt[]{5}\text{ = }(20)/(2)\text{ = 10 squared units} \end{gathered}`