Question

F (x) = √x -8. Find f -1 and its domain.

Answer 1

`f^(-1)(x)=(x+8)^2\text{ ; }x\text{ }\ge-8\text{ (option C)}`

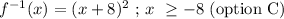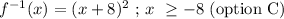
Step-by-step explanation:
`f(x)\text{ = }\sqrt[]{x}\text{ - 8}`

let y = f(x)

`y\text{ = }\sqrt[]{x}\text{ - 8}`

To get the inverse of f(x): first, we will interchange y and x. Then we will solve for y.

`\begin{gathered} In\text{tercahnge y and x:} \\ x\text{ = }\sqrt[]{y}\text{ - 8} \end{gathered}`

Add 8 to both sides:

`\begin{gathered} x\text{ + 8 = }\sqrt[]{y}\text{ - 8 + 8} \\ x\text{ + 8 = = }\sqrt[]{y} \\ \text{square both sides:} \\ (x+8)^2\text{ = (}\sqrt[]{y})^2 \\ y\text{ = }(x+8)^2\text{ } \\ \\ \text{Hence, }f^(-1)(x)\text{ = }(x+8)^2\text{ } \end{gathered}`

Domain are the inputs of a function. They are the x values.

The inverse of f(x) doesn't have a denominator.

The domain of an inverse functon is the range of the original function

Range (y values ) of original function was x ≥ -8

Hence, domain of this function is x ≥ -8

`f^(-1)(x)=(x+8)^2\text{ ; }x\text{ }\ge-8\text{ (option C)}`