Question

part A: A sample of a gas is in a sealed container. The pressure of the gas is 775 torr , and the temperature is 34 ∘C . If the temperature changes to 72 ∘C with no change in volume or amount of gas, what is the new pressure, P2 , of the gas inside the container?Part B: Using the same sample of gas ( P1 = 775 torr , T1 = 34 ∘C ), we wish to change the pressure to 7750 torr with no accompanying change in volume or amount of gas. What temperature T2 , in Celsius, is needed to reach this pressure?

Answer 1

Part A: the pressure of the gas is 869.78 torr.

Part B: 3075.4K (2802.25 °C) is needed to reach the pressure.

Step-by-step explanation:

Part A:

1st) The given information in the excecise is:

- P1: 775 torr

- T1: 34°C (307.15K)

- P2: this is what we have to calculate.

- T2: 72°C (345.15 K)

- Volume: constant.

2nd) To calculate the final pressure, it is necessary to use the Gay-Lussac's Law and replace the values:

`\begin{gathered} (P_1)/(T_1)=(P_2)/(T_2) \\ (775torr)/(307.15K)=(P_2)/(345.15K) \\ 2.52(torr)/(\degree C)=(P_2)/(345.15K) \\ 2.52(torr)/(\degree C)*345.15K=P_2 \\ 869.78torr=P_2 \end{gathered}`

Finally, the new pressure of the gas is 869.78 torr.

Part B:

1st) The given information in this case is:

- P1: 775 torr

- T1: 34°C (307.15K)

- P2: 7750 torr

- T2: this is what we have to calculate.

- Volume: constant.

2nd) To the final temperatue, we can also use the Gay-Lussac's Law and replace the values:

`\begin{gathered} (P_(1))/(T_(1))=(P_(2))/(T_(2)) \\ (775torr)/(307.15K)=(7,750torr)/(T_2) \\ 2.52(torr)/(K)=(7,750torr)/(T_(2)) \\ T_2=(7,750torr)/(2.52(torr)/(K)) \\ T_2=3,075.4K \end{gathered}`

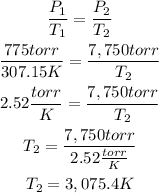
Finally, 3075.4K (2802.25 °C) is needed to reach the pressure.