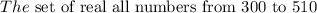Step 1
The domain of a function refers to all the values that go into a function. The domain of a function is the set of inputs accepted by the function. The output values are called the range.
Step 2
From the domain in this question, the number of days the crew has worked will determine the length of the road in miles. The domain is the independent quantity in a function. The number of days the crew has worked is independent of the length of the road. It does not depend on it.
Hence, the answer is;
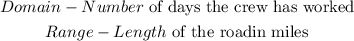
Step 3
Choose the most appropriate set of values. The crew can work for at most 70 days. Therefore;
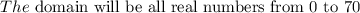
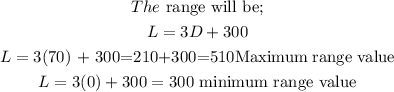
The range will be;