Question

A box, weighing 5.0 kg is pulled along a level floor at constant speed by a rope that makes an angle of 30 with the floor. If the force applied on the rope is 10 N. What is the coefficient of sliding friction between the box and the floor.

Answer 1

First, let's calculate the horizontal component of the force applied by the rope:

`\begin{gathered} F_x=F\cdot\cos(\theta)\\ \\ F_x=10\cdot\cos(30°)\\ \\ F_x=10\cdot0.866\\ \\ F_x=8.66\text{ N} \end{gathered}`

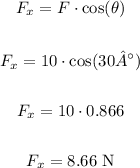
If the box has a constant speed, the net force is zero.

The net force is given by the horizontal component of the applied force minus the friction force:

`\begin{gathered} F_(net)=F_x-F_f\\ \\ 0=8.66-F_f\\ \\ F_f=8.66\text{ N} \end{gathered}`

Now, let's use the formula for the friction force, where, in this case, the normal force Fn will be equal to the weight force Fw:

`\begin{gathered} F_f=\mu\cdot F_N\\ \\ 8.66=\mu\cdot F_W\\ \\ 8.66=\mu\cdot m\cdot g\\ \\ 8.66=\mu\cdot5\cdot9.8\\ \\ \mu=(8.66)/(5\cdot9.8)=0.177 \end{gathered}`

Therefore the coefficient of sliding friction is 0.177.