We will have the following:
We will have the paralelogram ABCD and it has the diagonals AC congruent with BD.
Now, we will have that:
*Segment AB is parallel with segment CD [Given by definition]
*Segment AC is parallel with segment BD [Given by definition]
*We will have that the segment AD will be congruent with segment BC. [Opposite sides of a parallelogram are congruent]
*We then will have that segments AB will be congruent with AB, and segment DC will be congruent with DC. [Reflexive property.
*Then we will have:
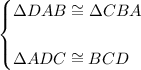
[We will have this by SSS congruence]
*Then:
![\begin{cases}<\text{DAB}\cong[This is directly derived from the previous point]<p></p><p>*Then we will have:</p>[tex]\begin{cases}<\text{DAB}+[This is that the angles DAB & CBA are supplementary, likewise angles ADC and BCD]<p></p><p>*And since congruent angles are equal we will have that:</p>[tex]\begin{cases}[Substitution]<p></p><p>*Then:</p>[tex]\begin{cases}2<\text{DAB}=180 \\ \\ 2<\text{ADC}=180\end{cases}]()
[Addition]
*Then we determine the value of each angle:

[Division]
*Then we will have that:
*Then we have proved that a parallelogram with congruent diagonals is a rectangle.