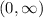To graph an exponential function:
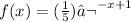
1. Use the equation to find few points in the graph:
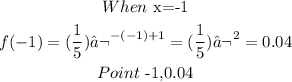
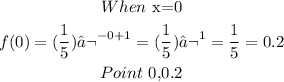

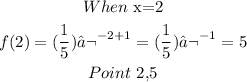
2. Identify the slope, using the points above you can see that the function is increasing (when x increases, y also increases)
3. Identify the end behavior:
As x increases y approaches to infinite
As x decreases y aproaches to 0, the horizontal asymptote is the x-axis
4. Graph:
Use the information you get in previous steps to graph:
5. Identify domain and range.
The domain of a function is the set of x-values for which the function is defined
The range of a function is the y-values that the function takes
For the given function:
Domain: All real numbers
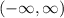
Range: All y greater than 0