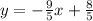Question

-6x-5y=3x-8 in slope intercept form step by step

Answer 1

Given the question in the image, the following are the solution steps to answer the question.

STEP 1: Write the given equation.

`-6x-5y=3x-8`

STEP 2: Write the general slope intercept form of the equation of a line

`\begin{gathered} y=mx+c \\ \text{where m is the slope, c is the y-intercept} \end{gathered}`

STEP 3: Write the given equation in slope-intercept form

Add 6x to both sides

`\begin{gathered} -6x-5y=3x-8 \\ -6x-5y+6x=3x-8+6x \\ -5y=9x-8 \end{gathered}`

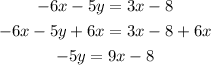
STEP 4: Divide both sides by -5

`\begin{gathered} (-5y)/(-5)=(9x-8)/(-5) \\ y=(9x-8)/(-5) \end{gathered}`

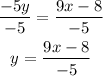
STEP 5: Write in the slope intercept form

`\begin{gathered} y=(9x-8)/(-5) \\ \text{Split into units} \\ y=(9x)/(-5)-((8)/(-5)) \\ \min us\text{ times minus becomes plus for the y-intercept} \\ y=-(9)/(5)x+(8)/(5) \end{gathered}`

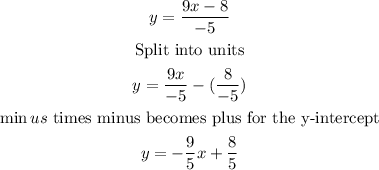
Hence, the slope intercept form is given as:

`y=-(9)/(5)x+(8)/(5)`