Question

A large bag of M&M candy has 178 red colored candy, 154 blue colored candy, 122 yellowcolored candy, and 93 green colored candy. Three pieces of candy are randomly chosen fromthe bag. What is the probability that all three candies are yellow?

Answer 1

The M&M bag has

R: 178 red colored candy

B: 154 blue colored candy

Y: 122 yellow colored candy

G: 93 green colored candy

The total number of candies in the bag are

`178+154+122+93=547`

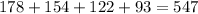
To calculate the probability for each colour you have to divide the number of candies of each colour by the total number of candies in the bag

`P(R)=(178)/(547)`
`P(B)=(154)/(547)`
`P(Y)=(122)/(547)`
`P(G)=(93)/(547)`

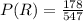
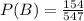
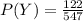
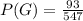
You have to calculate the probability of chosing three yellow candies:

`P(Y_{}\cap Y\cap Y)=P(Y)\cdot P(Y)\cdot P(Y)=3P(Y)=3\cdot((122)/(547))=(366)/(547)`

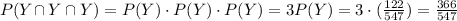
As percent

`(366)/(547)\cdot100=66.91`

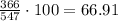
It is 66.91%