Question

Angelo drew a large square with an area of 100 square units. If he divided the large square into four congruent smaller squares, what is the side length of each of the small squares?5 units25 units10 units100 units

Answer 1

5 units

Step-by-step explanation

Step 1

find the length of the side

the area of a square is given by :

`\text{Area}=\text{ }side^2`

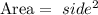
then Let

Area= 100 square units

replace and solve for side

`\begin{gathered} 100(un^2)=\text{ }side^2 \\ \text{square root in both sides} \\ √(100(un^2))=\text{ }√(side^2) \\ \text{side}=10\text{(un)} \end{gathered}`

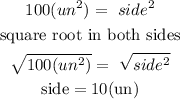
Step 2

now, the square is divided

now, to check the measure of the side length of each of the small squares we need divide the original side by 2

so

`\begin{gathered} \text{Side}_(small)=(side)/(2) \\ \text{replacing} \\ \text{Side}_(small)=(10)/(2) \\ \text{Side}_(small)=5 \end{gathered}`

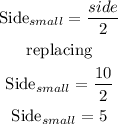
therefore, the answer is

5 units

I hope this helps you