Question

Trevor took big steps to find the length of the shadow of the big pine tree in front of the school. He estimated that the shadow was 16 yards long, or about 48 feet. He also stepped off the shadow cast by the two-story school building and estimated that it's shadow was 18 feet long. Trevor thinks the building is 24 feet tall. About how many feet tall is the big pine tree?

Answer 1

Trevor estimated that the building is 24 feet tal and its shadow is 18 feet long, therefore, we can see this as a right triangle. And we also have a pine tree whose shadow is 48 feet long.

In this cases, we consider the angles are the same, therefore, using trigonometry we can calculate the height of the tree as it follows

`\begin{gathered} \tan \theta=(opposite)/(adjacent) \\ \tan \theta=(24)/(18)=(H)/(48) \end{gathered}`

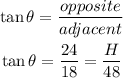
where H is the height of the tree, thus:

`\begin{gathered} (24)/(18)=(H)/(48) \\ H=48\cdot(24)/(18) \\ H=64 \end{gathered}`

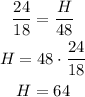
So, the big pine tree is about 64 feet tall,