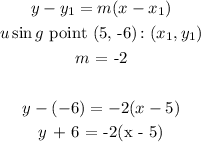y + 6 = -2(x - 5)
1st unknown: -6
2nd unknown: -2
3rd unknown: 5
Step-by-step explanation:
The given points: (5, -6) and (-1, 6)
To get the equation in point slope form, we will apply the formula:
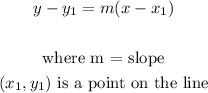
First let's find the slope of the line using the given points:
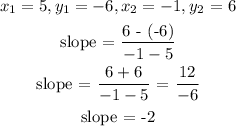
Next we pick any of the points and the slope to get point slope form: