Question

A) which hall reaches a greater maximum height?B) which ball is traveling at a faster average rate on the interval [0,2]?Explain your answers to part (a) and part (b).

Answer 1

Given the question in the image, the following are the solution steps to answer the question.

STEP 1: State the maximum height for the ball 1

The maximum height of the ball 1 is shown below:

STEP 2: State the maximum height for the ball 2

Given the function,

`-16t^2+40t+4`

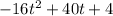
The graph is as seen below and the maximum point is indicated:

Maximum height is 29

Hence, the ball that reaches the greater maximum height is ball 2

STEP 3: Answer Part B

Average rate is calculated using the formula:

`(f(b)-f(a))/(b-a)`

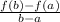
STEP 4: Find the average rate for ball 1

`\begin{gathered} a=0,b=2 \\ f(a)=2.5,f(b)=8 \\ rate=(8-2.5)/(2-0)=(5.5)/(2)=2.75 \end{gathered}`

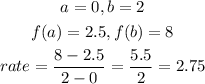
STEP 5: Find the average rate for ball 2

`\begin{gathered} a=0,f(a)=4 \\ b=2,f(b)=20 \\ rate=(20-4)/(2-0)=(16)/(2)=8 \end{gathered}`

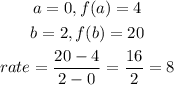
Since the average rate of change for ball 2 is more than that of ball 1, therefore ball 2 was travelling at a faster average rate on the given interval.