Given:
Two cards are drawn without replacement from a standard deck of 52 playing cards.
Required:
Find the probability of choosing a diamond for the second card drawn, if the first card, drawn with replacement, was a club.
Step-by-step explanation:
The total number of cards in the deck = 52
The number of diamonds cards = 13
The number of club cards = 13
The probability of an event is given by the formula:
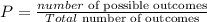
The probability that the first card is a club:
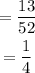
If the drawn first card is not replaced then the left card is = 51
The probability that the second card is a diamond :

Now the probability of choosing two cards, if the first is a club and the second is a diamond:
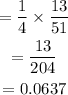
Final answer:
The required answer is 0.0637.