We have to evaluate cos(2θ), knowing that θ is in the first quadrant and in standard position P(u,v) = (3,4).
We can picture this as:
We can write the relation:
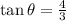
We now look at the identities to find cos(2θ):
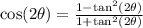
There are many identities for cos(2θ), but this is expressed in the information we already know, so we can solve as:
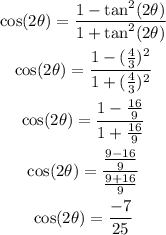
Answer: cos(2θ) = -7/25