Question

Use synthetic division to determine whether the first expression is a factor of the second. If it is, indicate the factorization. (If the first expression is not a factor of the second, enter DNE.)

Answer 1

Step-by-step explanation:

Given:

`\begin{gathered} Divisor:x-2 \\ Dividend:3x^4-6x^3-3x+6 \end{gathered}`

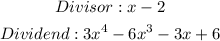
To find:

Use synthetic division to determine whether the first expression is a factor of the second

We'll follow the below steps to solve the given question;

Step 1: Write the coefficients of the dividend and write the constant term of the divisor with the opposite sign as seen below;

Step 2: Bring down the first coefficient of the dividend, multiply it by 2, and add the result to the next coefficient of the dividend as seen below;

Step 3: Multiply 0 by 2 and add the result to the next coefficient of the dividend;

Step 4: Multiply 0 by 2 and add the result to the next coefficient of the dividend;

Step 5: Multiply -3 by 2 and add the result to the next coefficient of the dividend;

We've completed the division and the coefficients are 3, 0, 0, -3, 0.

All the coefficients are the coffiecin