Question

Find the measure of angle yA. 19B. 22C.41D.120

Answer 1

We have that the sum of the internal angles of a triangle is 180°, then:

`\begin{gathered} \angle1+19+41=180 \\ \angle1+60=180 \\ \angle1+60-60=180-60 \\ \angle1=120 \end{gathered}`

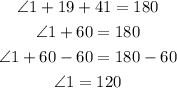
This is, angle 1 measures 120°. So, angle 1 and angle 2 are opposite angles at the vertex, so they have the same measure.

`\angle2=\angle1=120`

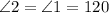
Angle 2 measures 120°.

Next, we know that the sum of the internal angles of a triangle is 180°, therefore the measure of angle y is:

`\begin{gathered} 38+\angle2+\angle y=180 \\ 38+120+\angle y=180 \\ 158+\angle y=180 \\ 158+\angle y-158=180-158 \\ \angle y=22 \end{gathered}`

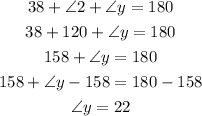
Angle y measures 22°.

Answer: B. 22°