Question

how would I answer part c. The table is N,number of weeks:0. 1. 2. 3. 4w(n),desired weight:183 181 179 177 175

Answer 1

Answer:

My desired weight is a linear function of time because it has a constant average rate of change per week. The slope (average rate of change) is -2

Step-by-step explanation:

Given:

the loss in weights per week

To find:

To fill in the blanks

For a function to be a linear function of time, it will have a constant rate of change

To get the slope, we will pick any two pairs of points from the table:

using points (0, 183) and (1, 181)

`\begin{gathered} m\text{ = }(y_2-y_1)/(x_2-x_1) \\ \\ x_1=0,y_1=183,x_2=1,y_2\text{ = 181} \\ m\text{ = }\frac{181\text{ - 183}}{1-0} \\ m\text{ = -2/1} \\ m\text{ = -2} \\ \\ Using\text{ points: \lparen1, 181\rparen and \lparen2, 179\rparen} \\ m\text{ = }(179-181)/(2-1) \\ m\text{ = -2} \end{gathered}`

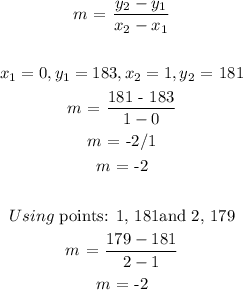
My desired weight is a linear function of time because it has a constant average rate of change per week. The slope (average rate of change) is -2