We have the next system of equations
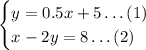
To solve the systme we must replace equation (1) in equation (2)
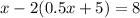
Simplifying,
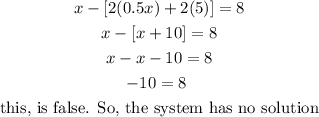
To graph the system we must graph equation (1) and (2)
- equation (1)
x-intercept: (-10, 0)
y-intercept: (0, 5)
-equation (2)
x-intercept
The graphs never intersect, we can confirm that the system has no solution.