Given:
The function is, f(x) = 3x+2.
The domain is, {-2, -1, 2}.
The objective is to find the range of the function.
If domain is, x = -2,
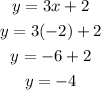
If domain is, x = -1,
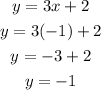
If domain is, x = 2,
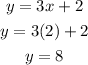
Thue, the domain is {-4, -1, 8}.
Hence, option (b) is the correct answer.