Question

Identify which process must be applied to the expression to come up with the given respective simplified version.

Answer 1

Let's breakdown the process to determine what among the 5 procedures was being applied to simplify the equations.

A.) From 6x + 9 = 4x - 3 to 2x + 9 = -3

By applying the inverse property of addition, we get,

`\text{ 6x + 9 = 4x - 3 }\rightarrow\text{ 6x + 9 - 4x = 4x - 3 -4x}`
`\text{ 2x + 9 = -3}`

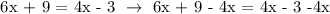
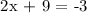
Thus, for 6x + 9 = 4x - 3 to become 2x + 9 = -3, we add -4x to both sides.

Number 4 is the procedure being applied.

B.) From -4(5x - 7) = -18 to 5x - 7 = 4.5

By applying the inverse property of multiplication, we get,

`\text{ -4(5x - 7) = }-18\text{ }\rightarrow\text{ }\frac{-4(5x\text{ - 7)}}{-4}\text{ = }(-18)/(-4)`
`\text{ 5x - 7 = 4.5}`

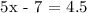
Thus, for -4(5x -7) = -18 to become 5x - 7 = 4.5, we multiply both sides by -1/4.

Number 1 is the procedure being applied.

C.) From 8 - 10x = 7 + 5x to 4 - 10x = 3 + 5x

We add -4 to both sides,

`\text{ (8 -10x) - 4 = (7 + 5x) - 4 }\rightarrow8\text{ - 10x - 4 = 7 - 5x - 4}`
`\text{ 4 - 10x = 3 + 5x}`

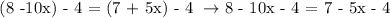
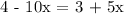
Thus, Number 5 is the procedure that must be applied.

D.) From (-5/4)x = 4 to 5x = -16

Applying the inverse property of multiplication, we get

`\text{ }(-5)/(4)x\text{ = 4 }\rightarrow\text{ (-}(5)/(4)x)(-4)\text{ =}4(-4)`
`\text{ 5x = -16}`

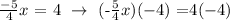
Thus, for (-5/4)x = 4 to become 5x = -16, we multiply both sides by -4.

Thus, Number 2 is the procedure the must be applied.

E.) From 12x + 4 = 20x + 24 to 3x + 1 = 5x + 6

Let's apply procedure Number 3 if it is the right one to be applied. We get,

`\text{ 12x + 4 = 20x + 24 }\rightarrow\text{ (12x + 4)(}(1)/(4))\text{ = (20x + 24)(}(1)/(4))`
`(12)/(4)x\text{ + }(4)/(4)\text{ = }(20)/(4)x\text{ + }(24)/(4)`
`\text{ 3x + 1 = 5x + 6}`

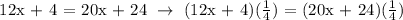
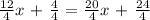
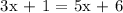
Thus, Number 3 is the procedure that must be applied.