Question

Which has the greater kinetic energy, a car traveling 30 km/hor a half as heavy car traveling at 60 km/h? (Hint: they do not have the same kinetic energy)

Answer 1

Given data:

* The velocity of the first car is 30 km/h

* The velocity of the second car is 60 km/h

Solution:

Let m is the mass of the first car.

As given mass of the second car is half as the first car.

Thus, the mass of the second car is,

`m^(\prime)=(m)/(2)`

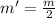
The kinetic energy of the first car is,

`K_1=(1)/(2)mv_1^2`

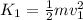
where v_1 is the velocity of the first car,

The velocity of the second car in terms of first car is,

`\begin{gathered} v_2=60\text{ km/h} \\ v_2=2*30\text{ km/h} \\ v_2=2v_1 \end{gathered}`

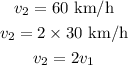
The kinetic energy of the second car is,

`K_2=(1)/(2)m^(\prime)v^2_2`

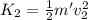
Substituting the known values,

`\begin{gathered} K_2=(1)/(2)*(m)/(2)*(2v_1)^2 \\ K_2=mv^2_1 \\ K_2=2K_1 \end{gathered}`

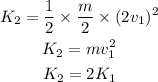
Thus, the kinetic energy of the second car with 60 km/h speed is twice the kinetic energy of the first car with 30 km/h speed.

Hence, the car with 60 km/h have greater kinetic energy than the car with speed of 30 km/h.