Question

A substance decays according to A=A0e−0.028t, where t is in hours and A0 is the initial amount. Determine the half-life of the substance. Round your answer to two decimal places, if necessary.

Answer 1

- The decay of the substance is governed by the following function:

`A=A_0e^(-0.0028t)`

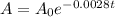
- We are asked to find the half-life of the substance.

- The half-life of the substance is the time it takes for the substance to decay from its original mass to half its original mass.

- Based on this definition, we can say that:

`A=(A_0)/(2)`

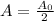
- Thus, we can find the half-life as follows:

`\begin{gathered} A=A_0e^(-0.0028t) \\ \\ \text{ Put }A=(A_0)/(2) \\ \\ (A_0)/(2)=A_0e^(-0.0028t) \\ \\ \text{ Divide both sides by }A_0 \\ \\ (1)/(2)=e^(-0.0028t) \\ \\ \text{ Take the natural log of both sides} \\ \\ \ln(1)/(2)=\ln e^(-0.0028t) \\ \\ \ln(1)/(2)=-0.0028t \\ \\ \text{ Divide both sides by -0.0028} \\ \\ \therefore t=(1)/(-0.0028)\ln(1)/(2) \\ \\ t=247.55256...\approx247.55\text{ hours} \end{gathered}`

Final Answer

The half-life is 247.55 hours