Let speed of plane be "x" and speed of wind be "w".
• With the wind, the speed of plane becomes:
x + w
• Against the wind, the speed of plane becomes:
x - w
We know D = RT, where
D is distance
R is rate
T is time
Going against the wind, it takes 7 hours, so we can write:
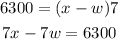
Going with the wind, it takes 6 hours, thus:

Let's multiply the first equation by 6 and the second equation by 7:
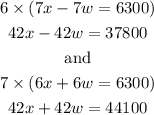
Adding the two new equations, we can eliminate w and solve for x:
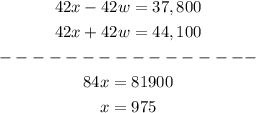
We can use this value of x and put it into the first equation and solve for w:
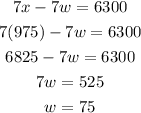
Thus,
Answer
Speed of Plane = 975 km/hr
Speed of Wind = 75 km/hr