We will investigate how to interpret a statement and translate it into an inequality. Then we will go ahead with a plot to represent the inequality.
We are given the following statement:
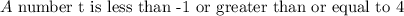
We see that we are looking at a one dimensional inequality i.e the inequality involves a single variable " A number t ".
We will develop an inequality to express the domain of the variable ( t ) by looking for certain keywords in the statement given. The general inequality translation rules are employed:

We wil use the above rules and translate the given statement into a mathematical inequality:

We can plot the above inequality as a one dimensional number line as follows:
Each box represents a magnitude of one unit!
The right open circle should be filled due to the equal to " = " sign!