Question

Mathematics, helpPart CNext, the archeologist dilates the new image of the bone fragment using a scale factorof 1.5 and a center of dilation at the origin. What are the new coordinates of thevertices? Write the coordinate pairs.A": ___________B": ___________C": ___________D": ___________Part DBy what factor does the dilation cause the perimeter of the image to be multiplied? The perimeter of the image is multiplied by a factor of ___?By what factor does the dilation cause the perimeter of the image to be multiplied? The areaof the image is multiplied by a scale factor of ___?Part EExplain why the area and the perimeter are not both multiplied by the same factor.

Answer 1

PART C:

A'(-6,6)

B'(-3,6)

C'(-3,3)

D'(-6,3)

PART D:

The perimeter of the image is multiplied by a factor of 3

The area of the image is multiplied by a factor of 9

PART E:

Since the perimeter measures the distance around the outer edge of the figure and the area measures the space inside the figure, they will have different measures since they are measuring different things. That's why they are multiplied by different factors.

Step-by-step explanation:

From the given graph above, we can see that the coordinates of the vertices of ABCD are A(-4, 4), B'(-2, 4), C'(-2, 2), and D'(-4, 2).

PART C:

If ABCD is dilated by a scale factor of 1.5 a center of dilation at the origin, the coordinates of the vertices of the image A'B'C'D', will be;

`\begin{gathered} A^(\prime)[1.5(-4),1.5(4)]\rightarrow A^(\prime)(-6,6) \\ \\ B^(\prime)[1.5(-2),1.5(4)]\rightarrow B^(\prime)(-3,6) \\ \\ C^(\prime)[1.5(-2,),1.5(2)]\rightarrow C^(\prime)(-3,3) \\ \\ D^(\prime)[1.5(-4),1.5(2)]\rightarrow D^(\prime)(-6,3) \end{gathered}`

See the graph of A'B'C'D' below;

PART D:

We can see that;

Length of pre-image(l)= 2

Width of pre-image(w) = 2

Let's go ahead and determine the perimeter of the pre-image as seen below;

`\begin{gathered} Perimeter\text{ of the pre-image}=2(l+w) \\ \\ \begin{equation*} 2(2+2) \end{equation*} \\ \\ =2(4) \\ \\ =8 \end{gathered}`

From the graph of the image, we can see that;

Length of image = 6

Width of image = 6

Let's go ahead and determine the perimeter of the image as seen below;

`\begin{gathered} Perimeter\text{ of the image}=2(6+6) \\ \\ =2(12) \\ \\ =24 \end{gathered}`

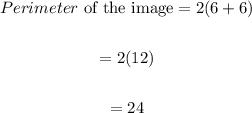
Since the perimeter of the pre-image is 8 and that of the image is 24, we can see that the perimeter of the image is multiplied by a factor of 3.

Let's also find the area of the pre-image as seen below;

`\begin{gathered} Area\text{ of the pre-image}=l*w \\ \\ =2*2 \\ \\ =4\text{ square units} \end{gathered}`

Area of the image will be;

`\begin{gathered} Area\text{ of the image}=l*w \\ \\ =6*6 \\ \\ =36\text{ square units} \end{gathered}`

Since the area of the pre-image is 4 and that of the image is 36, we can see that the area of the image is multiplied by a factor of 9.

PART E:

Since the perimeter measures the distance around the outer edge of a shape and area measures the space inside a shape, they will have different measures since they are measuring different things. That's why they have different scale factors.