Question

On a dogleg golf hole, one golfer hits the ball 270 yards and then another 170 yards to reach the green. The angle between the two hits is equal to 100 degrees. How far would the golfer have to originally hit the ball for it to go directly to the same position on the green?150.463 yards 106.746 yards 343.134 yards 117,740.903 yards

Answer 1

We have the following triangle to describe this situation

The distance between the golfer and the green is represented by x. We can solve this problem using the cosine law. By the cosine law, we have the following relation between those measures

`x^2=270^2+170^2-2\cdot270\cdot170\cdot\cos (100^o)`

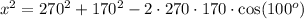
Solving for x, we have

`\begin{gathered} x^2=270^2+170^2-2\cdot270\cdot170\cdot\cos (100^o) \\ x^2=72900+28900-91800\cos (100^o) \\ x^2=117740.90271\ldots \\ x=\sqrt[]{117740.90271\ldots} \\ x=343.133942812\ldots \\ x\approx343.134 \end{gathered}`

The distance between the golfer and the green is 343.134 yards.