Question

Suppose babies born after a gestation period of 32 to 35 weeks have a mean weight of 3000 grams and astandard deviation of 1000 grams while babies born after a gestation period of 40 weeks have a mean weight of3400 grams and a standard deviation of 515 grams. If a 35-week gestation period baby weighs 3425 gramsand a 40-week gestation period baby weighs 3825 grams, find the corresponding Z-scores. Which babyweighs more relative to the gestation period?

Answer 1

D. The baby born in 40 weeks weighs more relatively since its z-score, 0.83 is larger than the z-score of 0.43 for a baby born in week 35.

Explanation:

The z-score is calculated using the formula:

`z=(X-\mu)/(\sigma)\text{ where }\begin{cases}X=\text{Raw Score} \\ \mu=\text{Mean} \\ \sigma=\text{Standard Deviation}\end{cases}`

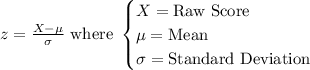
For the baby born after a 35-week gestation period.

• Weight = 3425 grams

,

• Mean = 3000 grams

,

• Standard Deviation = 1000 grams

The z-score is calculated below:

`z=(3425-3000)/(1000)=(425)/(1000)=0.425\approx0.43`

The z-score is 0.43 correct to 2 decimal places.

For the baby born after a 40-week gestation period.

• Weight = 3825 grams

,

• Mean = 3400 grams

,

• Standard Deviation = 515 grams

The z-score is calculated below:

`z=(3825-3400)/(515)=(425)/(515)\approx0.83`

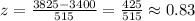
The z-score is 0.83 correct to 2 decimal places.

Thus, we conclude that:

The baby born in 40 weeks weighs more relatively since its z-score, 0.83 is larger than the z-score of 0.43 for a baby born in week 35.

Option D is correct.