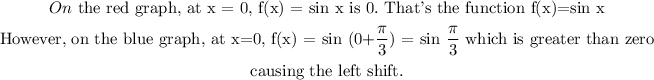Question

Describe the transformation of f(x) = sin x to g(x) = sin (x + 5).O A. Ax) is shifted į units up.B. f(x) is shifted units down.C. f(x) is shifted units to the left.O D. f(x) is shifted units to the right.

Answer 1

As you can see the red graph depicts sin x while the blue depicts sin (x+(pi/3))

The effect of the pi/3 to the original function is to simply shift the graph leftwards.

The usual f(x) = sin x graph is a periodic function graph that oscillates between 1 and -1. That's the red graph.

`\begin{gathered} On\text{ the red graph, at x = 0, f(x) = sin x is 0}.\text{ That's the function f(x)=sin x} \\ \text{However, on the blue graph, at x=0, f(x) = sin (0+}(\pi)/(3)\text{) = sin }(\pi)/(3)\text{ which is greater than zero} \\ \text{causing the left shift.} \end{gathered}`