Question

A student drove to the university from her home and noted that the odometer on her car increased by 16.0 km. The trip took 19.0 min.(a) What was her average speed?km/h(b) If the straight-line distance from her home to the university is 10.3 km in a direction 25.0° south of east, what was her average velocity?km/h (25° S of E)(C) She returned home by the same path. The total time to leave home, travel to the university, and return home was 7 h 30 min. What were her average speed and velocityfor the entire trip?average speedkm/haverage velocitykm/h

Answer 1

a. 50.53 km/h

b. 32.53 km/h (in the direction of 25˚ S of E)

c. 4.27 km/h

d. 0 km/h

Explanation:

a.

We have that the speed is given by the following formula:

`\begin{gathered} s=(d)/(t) \\ \text{ where s is the speed, d is the distance and t is the time} \end{gathered}`

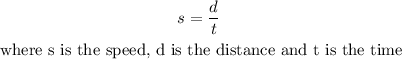
We know that the equivalence between minutes and hours is that 60 minutes equals 1 hour, therefore:

`s=\frac{16\text{ km}}{19\text{ }\min\frac{1\text{ h}}{60\text{ min}}}=50.53\text{ km/h}`

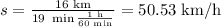
b.

The velocity is given by the following formula

`\begin{gathered} v=(\Delta x)/(t) \\ \text{replacing:} \\ v=\frac{10.3\text{ km-}0\text{ km}}{19\text{ }\min \frac{1\text{ h}}{60\text{ min}}}=32.53 \end{gathered}`

c.

The total distance traveled would then be two 16 kilometers, that is 32 km and the time would be 7.5 hours. we replace

`s=\frac{32\text{ km}}{7.5\text{ h}}=4.27\text{ km/h}`

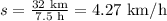
d.

Since it starts in the same place that it ends, the displacement is equal to 0, therefore, the velocity is equal to 0 km/h