Question

use the formula for future value, A=P( 1 + rt ) and elementary algebra to find the missing quantity. A = $2,556r = 6% t = 7 years

Answer 1

Given:

A = $2,556

r = 6% = 0.06

t = 7 years

Let's use the formula A=P(1 + rt) to find the missing quantity.

Where:

A is the final amount

P is the Principal amount

r is the interest rate

t is the time in years

From the given values, the missing quantity is the principal P.

Hence, let's solve for P.

Since we are to solve for P, let's rewrite the formula for P.

Divide both sides of the equation by (1 + rt):

`\begin{gathered} (A)/((1+rt))=(P(1+rt))/((1+rt)) \\ \\ (A)/((1+rt))=P \\ \text{ Rewrite the equation:} \\ P=(A)/((1+rt)) \end{gathered}`

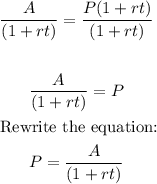
Substitute 2556 for A, 0.06 for r, and 7 for t in the equation:

`P=(2556)/(1+0.06\ast7)`

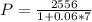
Solving further:

`\begin{gathered} P=(2556)/(1+0.42) \\ \\ P=(2556)/(1.42) \\ \\ P=1800 \end{gathered}`

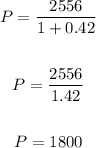
Therefore, the missing quantity, P, is 1800.

The Principal amount, P, is $1,800

ANSWER:

$1800