We know that
• The acceleration is 3.0 m/s^2.
Using Newton's Second Law, we have
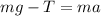
The two forces involved are the weight of the elevator and the tension force. Let's solve got T.
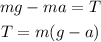
The mass of the elevator is m = 5000kg, g = 9.8 m/s^2, and a = 3 m/s^2.
![\begin{gathered} T=5000\operatorname{kg}(9.8((m)/(s^2))-3((m)/(s^2))) \\ T=5000\operatorname{kg}\cdot6.8((m)/(s^2)) \\ T=5000\operatorname{kg}\cdot7((m)/(s^2)) \\ T=35,000N \end{gathered}]()
Therefore, the tension in the cable is 35,000 N.