Question

1. Roger tosses a ball straight upward at a velocity of +32m/s, calculate the maximum height of the ball and the time to get to the maximum height.

Answer 1

Given data:

* The initial velocity of the ball is 32 m/s.

* The acceleration due to gravity of the ball is,

`g=9.8ms^(-2)`

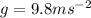
* The final velocity of the ball is 0 m/s.

Solution:

(a). By the kinematics equation, the maximum height of the ball is,

`v^2-u^2=-2gh`

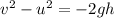
where v is the final velocity, u is the initial velocity, h is the maixmum height, and g is the acceleration due to gravity,

Substituting the known values,

`\begin{gathered} 0-(32)^2=-2*9.8* h \\ 1024=19.6h \\ h=(1024)/(19.6) \\ h=52.24\text{ m} \end{gathered}`

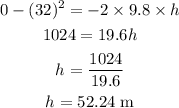
Thus, the maximum height of the ball is 52.24 m.

(b). By the kinematic equation, the time taken by the ball to reach the maximum height is,

`\begin{gathered} v-u=-gt \\ 0-32=-9.8* t \\ 32=9.8t \\ t=(32)/(9.8) \\ t=3.26\text{ s} \end{gathered}`

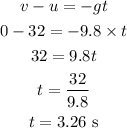
Thus, the time taken by the ball to reach the maximum height is 3.26 seconds.