Mickey mows 1/3 of a lawn in 10 minutes.
So, his rate = (1/3) / 10 = 1/30 lawn/min
Minnie mows 1/4 of the lawn in 6 minutes.
So, his rate = (1/4) / 6 = 1/24 lawn/min
compare the fractions:
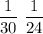
Make the denominator are equal so, the common denominator is ( 30 x 24 = 720 )
So,
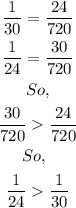
So, the rate of Minnie is faster than Mickey
This is because the rate of Minnie is greater than the rate of Mickey
So, the student reasoning is not correct