Question

Tell whether the table represents an exponential function. If so write a rule for the function X -2 -1 0 1 2 Y 25 5 1 1/5 1/25

Answer 1

Given:

In a table,

x values are -2, -1, 0, 1, 2.

y vales are 25, 5, 1, 1/5, 1/25

The objective is to prove whether the table values represents an exponential function or not. And also to find the rule for the function.

Consider the x values in the given table. The difference between each value will be,

`\begin{gathered} x_2-x_1=-1-(-2)_{} \\ =1 \end{gathered}`

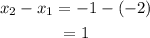
Similarly,

`\begin{gathered} x_3-x_2=0-(-1) \\ =1 \end{gathered}`

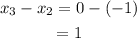
Thus, the values of x are constantly increasing with positive 1 unit.

Consider the y values in the given table.

Te ratio between each term will be,

`\begin{gathered} (y_2)/(y_1)=(5)/(25) \\ =(1)/(5) \end{gathered}`

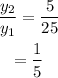
Similarly,

`(y_3)/(y_2)=(1)/(5)`

Thus, there is a common ratio between each values of y in the table.

So, it is clear with the common ratio, that the given table value is an exponential functiton.

Now, the rule for the function can be written as,

`y=a\cdot b^x`

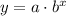
Consider the coordinate, (x,y) = (0,1) and substitute in the above formula.

`\begin{gathered} 1=a\cdot b^0 \\ 1=a\cdot1 \\ a=1 \end{gathered}`

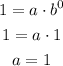
Consider another coordinate (1, 1/5) and the value of a. Substitute the obtained values in the general formula.

`\begin{gathered} (1)/(5)=1\cdot b^1 \\ b=(1)/(5) \end{gathered}`

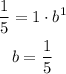
Now, substitue only the value of a and b in the general formula.

`\begin{gathered} y=1\cdot((1)/(5))^x^{} \\ y=((1)/(5))^x \end{gathered}`

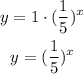
Hence, the rule for the given exponential function is y=(1/5)^x.