Step-by-step explanation:
Given;
We are given the triangle ABC on the coordinate plane with the following coordinates;
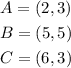
Required;
We are required to reflect ABC across the y-axis and then identify the coordinates of C' after the reflection.
Step-by-step solution;
The rule for reflecting across the y-axis is to make the x-coordinate negative and leave the y-coordinate untouched after the reflection.
Thus for the point C, the rule will have the following effect;
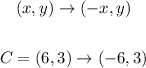
Therefore,
ANSWER:
The coordinates of C' after the reflection across the y-axis is;
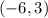
Option B is the correct answer