Question

-Finding the next terms of an arithmetic sequence with integersThe first three terms of an arithmetic sequence are as follows.-1, 2, 5

Answer 1

8 and 11

Explanations:

Given the sequence of numbers -1, 2, 5 __, __, you are to find the next two terms of the sequence.

The sequence is an arithmetic sequence and the nth term of an arithmetic sequence is expressed as:

`T_n=a+(n-1)d`

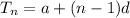
where:

• a is the, first term

,

• n is the, number of terms

,

• d is the ,common difference

From the given sequence;

a = -1

d = 2 - (-1) = 5 - 2

d = 3

For the fourth term, n = 4.

`\begin{gathered} T_4=-1+(4-1)\cdot3 \\ T_4=-1+3(3) \\ T_4=-1+9 \\ T_4=8 \end{gathered}`

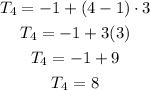
For the fifth term, n = 5;

`\begin{gathered} T_5=-1+(5-1)\cdot3 \\ T_5=-1+4(3) \\ T_5=-1+12 \\ T_5=11 \end{gathered}`

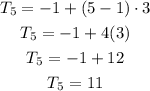
Therefore the next two terms of the given arithmetic sequence are 8 and 11