Question

X^2+y-9=0For the given equation, list the intercepts and test for symmetry.

Answer 1

We are given the following function:

`x^2+y-9=0`

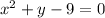
We are asked to determine the intercepts of the function.

To determine the y-intercept we will set "x = 0", we get:

`(0)^2+y-9=0`

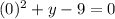
Now, we solve the operations:

`y-9=0`

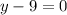
Now, we add 9 to both sides:

`y=9`

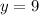
Therefore, the y-intercept is located at "y = 9".

To determine the x-intercept we will set "y = 0":

`x^2+0-9=0`

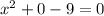
Now, we solve the operations:

`x^2-9=0`

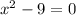
Now, we add 9 to both sides:

`x^2=9`

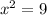
Taking the square root to both sides_:

`x=√(9)`

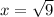
Solving the operations:

`x=\pm3`

This means that there are two x-intercepts:

`\begin{gathered} x=3 \\ x=-3 \end{gathered}`

To test for symmetry with respect to the y-axis we will substitute "x" for "-x" if we get the same function then there is symmetry with respect to "y".

`(-x)^2+y-9=0`

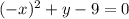
Solving we get:

`x^2+y-9=0`

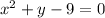
Since we got the same function this means that there is symmetry with respect to the y-axis.

To determine if there is symmetry with respect to the x-axis we will substitute the value of "y" for "*-y":

`x^2+(-y)-9=0`

Now, we solve the operations:

`x^2-y-9=0`

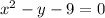
Since we get a different function there is no symmetry with respect to the x-axis.

To determine if there is symmetry with respect to the origin we will substitute "x" and "y" for "-x" and "-y":

`(-x)^2+(-y)-9=0`

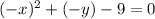
Solving the operations:

`x^2-y-9=0`

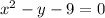
Since we didn't get the same function there is no symmetry with respect to the origin.