Given the equation:
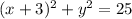
The given equation represents the equation of the circle
The general equation of the circle is:

Where r is the radius and the center at the point ( h , k )
So, compare the given equation to the general form, we will get:
![\begin{gathered} r^2=25 \\ r=\sqrt[]{25}=5 \\ \text{Center}=(h,k)=(-3,0) \end{gathered}](https://img.qammunity.org/2023/formulas/mathematics/college/ysdphlc2w3vf79uzvwrfzpgh4z8wot7gq8.png)
The graph of the given equation is as the following image: