Three or more points are collinear (belong to the same line), if slope of any two pairs of points is same. We can compute the slope for the the given points. The slope formula is given by

where, in our case,
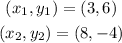
If we substitute these values into the slope formula, we have
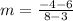
which gives
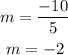
Now, we can compute the slope for the other couple of coordinates. If we take
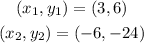
and we substitute these values into the slope formula, we have
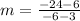
which gives
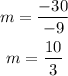
By comparing both slopes, we can see they are different. If the three points lie on a line, the slope must be the same. Then, these points do not belong to a straight line.