We have the following operation with two binomials:

And we have to find the result of the subtraction, and then graph that result.
To find the result of the subtraction, we can proceed as follows:
1. Multiply the second binomial by -1:

2. Add - algebraically - the like terms:
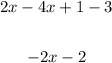
Therefore, the result for the subtraction is -2x - 2:
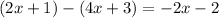
3. Now, to graph the result of the binomial, we can see that we have a line in the slope-intercept form:

And we know that b is the y-intercept of the line, that is, the line passes through the point (0, -2). The y-intercept is a point where the line passes through the y-axis when x = 0. We also can see that the slope of the line is negative, m = -2.
4. We can find the x-intercept so that we can graph the line easily using the intercepts. We know that the x-intercept is the point where the line passes through the x-axis when y = 0. Then we have to set it to zero y in the resulting equation above:

Therefore, the x-intercept is (-1, 0).
5. Now we can graph the resulting line by using the two resulting points: (-1, 0) and (0,-2). Then we have:
Therefore, in summary, we have that:
• The result of subtracting both binomials is ,-2x - 2
,
• And the graph of this result is (a line):